Gemini Puzzles- Create a Headache for Order of Operations!

OK, the MTBoS has really influenced the way I approach teaching and assigning problems.  Coming back from Christmas Break, I needed a different way to get students thinking about math again, and found that thanks to Math = Love in the form of Gemini Puzzles.

What are Gemini Puzzles?  In short, they are equality statements that are missing any mathematical symbols. Here is an example:

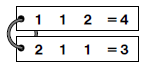
The thing I love about this is- I put these two on the whiteboard for students to see when they first walk in and instead of asking what we are doing today or talking about what they did last night, they started thinking about what was going on.  The other great thing, I didn’t have to tell them what to do, they started playing around with the numbers to get it done.

When I asked my students what they were doing, there was a common theme:

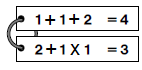
Which is great until I ask students about the name of the problem, Gemini.  I then get answers about twins and I tell them that’s relevant to the problem.  They are given two equivalence statements, and they have to be twins.  In other words, anything they add to one statement has to be the exact same as what they add to the second.

Then students start working in a stream of consciousness and they have this as an answer:

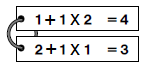
It doesn’t take too long for a student to say that there’s problem with this.  Students are thinking “one plus one is two, times two is four”, but they are not properly showing that.  Then the talk of Order of Operations hits, and students realize the first statement is only 3.  By throwing in another set of symbols they find the correct solution:

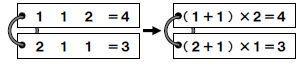
By providing students with two equivalence statements that need the exact same symbols to make them true, you are giving students a reason to think about the order of operations and how they interact.  I have loved listening to student talk when working on these, and I even had students proudly come up to me with different solutions to a problem.  They are amazed that there can be different ways to implement mathematical operations and get the same result (see if you can find which one they discovered!)

Gemini Puzzles are a great activity for the classroom, I know I’ll be using them from now on, I hope you enjoy them as well.

I currently have a range of students from 6th to 12th grade in my classes, with various mathematical skills ranging from 4th to 12th+, and these puzzles have engaged and challenged them all.  It’s a great thing to see.

Roll ‘Em Out, Line ‘Em Up!

Order of Operations:

Using mathematical operations, grouping symbols and equality symbols, create as many mathematical equations as you can using the numbers:

6  5  1  4  1  3  6

Subtraction That Makes Sense

One thing that still puzzles me is that I have 8th grade students who are fluent in only one mathematical operation, Adding.  Many students can only Multiply single digit numbers, and I have not come across an 8th grade class who says they like division.  Even when I start looking at “basic” math skills, many of my students commonly make mistakes subtracting- and it’s because they are using an algorithm they memorized, regrouping.

I started really digging at my students’ understanding of the subtraction algorithm to better understand where the misconceptions are based and found that they basically understood the rules they were to apply but did not understand why they did them.  This led to many mistakes involving place value and positive or negative numbers.  Equally surprising is that naturally students wanted to solve the problem in a totally different way.  I know many of you are familiar with various Subtraction methods, and this is the one that my students connected with.

My students naturally wanted to work from left to right on this problem, as well as indicate negative values when they performed the subtraction operation.  How can this be?  How does it magically work?  For students to be able to answer how it works, you will need to make sure they understand the underlying mathematics involved.  Do they understand place values, regrouping, the concept of negative values?  When watching Subtraction as a Confidence Builder, I really like how she allows her students to evaluate and use different methods, but even though they approach the problem in a similar fashion to my students, they still require that students work from right to left, and have to work “back up” their partial differences to find their answer.  This could be alleviated by allowing students to think of the problem naturally, and allowing students to add naturally instead of the per-described algorithm we all know.

From a mathematical standpoint, this approach to addition really illustrates the knowledge of place value as well as showing students where regrouping occurs in the traditional algorithm.  Notice the 150 line where we add 7 10’s and 8 10’s.  Students understand the concept of money and will tell you that 7 dimes plus 8 dimes is $1.50, and this carries over naturally into this type of addition method.

When I first saw this type of procedure for addition, I had some questions.  Is there some magical rule about starting in the left or right?  When you count your change, does it matter if you start with pennies first or quarters?  Realistically which do you normally count first?  Which way makes your total easier to find?  The answers to each of these changes on the individual who answers them, and that should also be encouraged when looking at initial addition.  There is no magical rule, only one imposed by your curriculum.

When we look back to subtraction, would it make more sense to really examine negative numbers when we introduce this to students?  My 5 year old son can understand if he has 4 monster trucks and I want to take away 5, that I can’t do that.  He will tell me that he needs one more truck to take away five.  He is thinking about positive and negative integers in Kindergarten, and needs to be encourage to do so.  Tell our younger students that they are doing Algebra, and that they are dealing with negative integers.  Many of our students will surprise you with their understanding.  If they are exposed to these concepts at a young age they will not struggle down the road in 8th grade when we try to add 8 + -3.  They will forget about the rules and understand what is being asked.  Maybe even some of them will quit asking me if it is OK to rewrite the problem vertically.